Modeling Resonant Inelastic X-ray Scattering using the Density Matrix Renormalization Group: Advancing the State of the Art
In quantum materials there is often a delicate balance between the 1023 electrons and ions that make up the material, and this gives rise to a diverse set of fascinating properties such as high-temperature superconductivity and colossal magnetoresistance. A crucial first step in understanding a material is the determination of how its magnetic, charge, and orbital degrees of freedom are excited by external stimuli. This is where inelastic scattering experiments such as resonant inelastic x-ray scattering (RIXS) come into play.
In a typical inelastic scattering experiment, particles (e.g., neutrons or photons) are bounced off of a material of interest. Depending on the energy of the particle, a significant amount of its kinetic energy and momentum can be transferred to the sample during the scattering process. By tracking the amount of energy/momentum lost by each particle, scientists can reconstruct how it might have interacted with the sample to learn something new about the material. The process is like throwing a rock off the end of a dock and watching how water waves form to determine the location of a nearby dock’s legs. RIXS makes use of high-energy x-rays, which makes it simultaneously sensitive to all of the magnetic, orbital, and charge degrees of freedom, and therefore a powerful experimental tool. RIXS’s versatility comes at a cost, however; its spectra are often complex and difficult to interpret without the aid of advanced theoretical modeling.
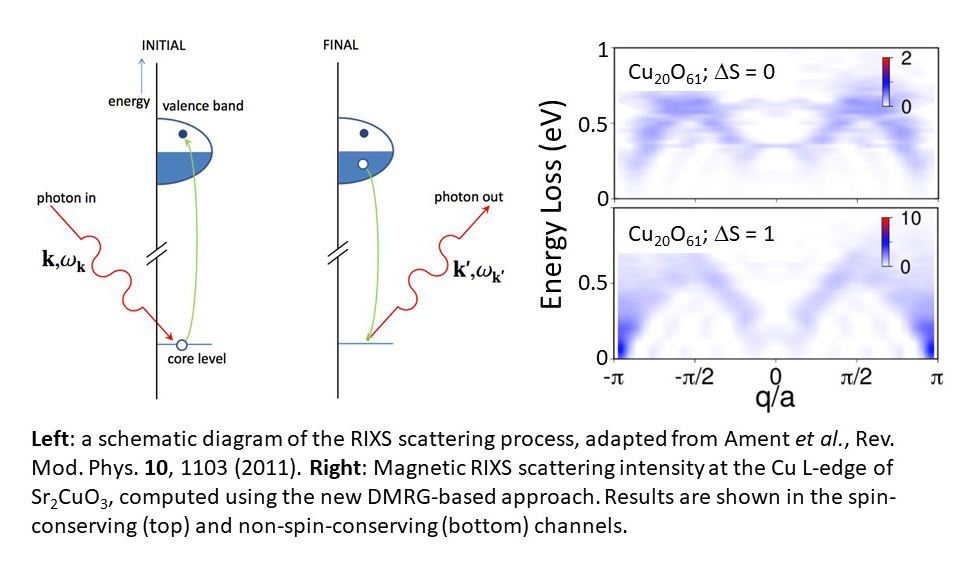
Until recently, the standard technique for modeling RIXS was exact diagonalization (ED), where one approximates the material by solving a very small cluster meant to represent the infinite system. Exact diagonalization is limited by a rapid growth in memory requirements, which severely limits the size of the problem that can be addressed and so a trade-off occurs between making the system as large as possible while still being trackable. In a paper published this week in Scientific Reports, a UT team lead by physicists Elbio Dagotto and Steve Johnston describe a new advanced numerical algorithm based on the Density Matrix Renormalization Group (DMRG) method, which overcomes this limitation in a particular class of low-dimensional materials.
DMRG is the most powerful method for computing the properties of one-dimensional systems but it is not often used to model excited properties measured in RIXS experiments. In their paper, the UT team extended this method to fill this gap. Importantly, DMRG is a CPU intensive algorithm, which shifts the computational bottleneck from the memory requirements associated with ED to the availability of CPUs. With this bottleneck cleared, the team was able to compute the RIXS spectra for systems up to four times larger than what has been accessible to ED. Given the rapid growth of leadership class supercomputing, the new algorithm opens the door even larger systems and new problems that could not be addressed previously.
Dagotto and Johnston’s work was supported by the U.S. Department of Energy, with computational resources from UT and Oak Ridge National Laboratory through the Joint Institute for Computational Sciences.
The article “Computing Resonant Inelastic X-Ray Scattering Spectra Using The Density Matrix Renormalization Group Method” by A. Nocera, U. Kumar, N. Naushal, G. Alvarez, E. Dagotto, and S. Johnston has been published in Scientific Reports, an open access journal, and is free to read for anyone (https://www.nature.com/articles/s41598-018-29218-8).